শ্রেণী- দশম ; অধ্যায় – ত্রিকোণমিতিক অনুপাত এবং ত্রিকোণমিতিক অভেদাবলি ; কষে দেখি 23.2
কষে দেখি 23.2 Class 10 অংকের সূচিপত্র:-
কষে দেখি 23.2 Class 10 এর অংকের সমাধান গুলি ভালোভাবে বোঝার জন্যে কিছু উপদেশঃ
এই কষে দেখি 23.2, পশ্চিমবঙ্গ মধ্যশিক্ষা পর্ষদ | WBBSE এর অন্তর্গত দশম শ্রেণি|Class 10 এর 23 নম্বর অধ্যায়|Chapter 23, ত্রিকোণমিতিক অনুপাত এবং ত্রিকোণমিতিক অভেদাবলি | Trigonometric Ratio and Trigometric Identities এর দ্বিতীয় অনুশীলনী।
এই কষে দেখি 23.2 Class 10 এর অঙ্ক গুলি বোঝার জন্যে যে যে বিষয় জানতে হবে তা আলোচনা করা হলো–
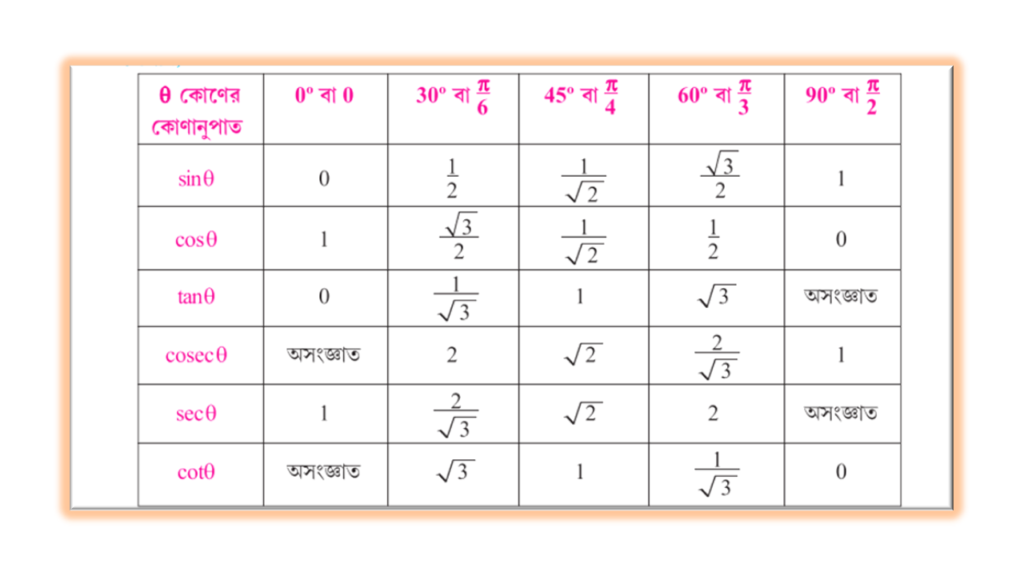
এই কষে দেখি 23.2 Class 10 এর অংকগুলি করার জন্যে কোণ হিসাবে \(\sin \theta, \cos \theta , \tan \theta, \csc \theta, \sec \theta, \cot \theta\) এর মান আমাদের জানতে হবে, যা নিম্নরূপ –
আগামিতে এই কষে দেখি 23.2 Class 10 এর অংক গুলির সমাধানের প্রয়োজন হলে কি করবে?
| কষে দেখি 23.2 Class 10 এর এই কষে দেওয়া অংক গুলি তোমাদের যদি আগামিতে আবার প্রয়োজন হয় তাহলে তোমরা Google এ গিয়ে Search করবে- কষে দেখি 23.2 Class 10 তারপর |
কষে দেখি 23.2 Class 10|Koshe Dekhi 23.2 Class 10
1.আমাদের বাড়ির জানালায় একটি মই ভূমির সঙ্গে 60° কোণে রাখা আছে। মইটি 2√3 মিটার লম্বা হলে আমাদের ওই জানালাটি ভূমি থেকে কত উপরে আছে ছবি এঁকে হিসাব করে লিখি।
সমাধানঃ-
জানালাটি ভূমি থেকে উপরে আছে
= AB মিটার.
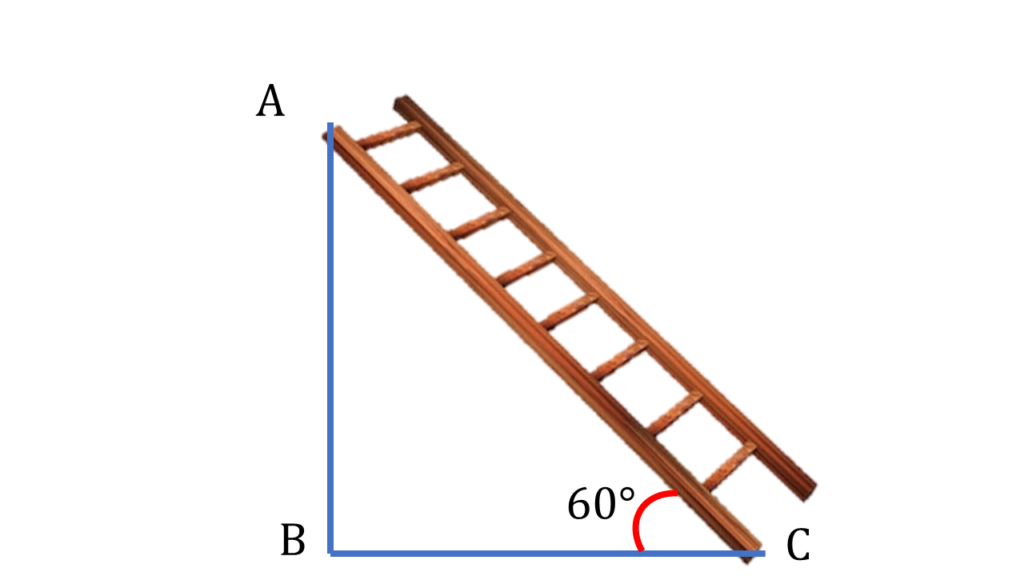
AC = মই এর দৈর্ঘ্য = 2√3 মিটার.
সমকোণী ▲ABC তে,
| \(\frac{AB}{AC} = \sin60°\) |
| বা, \(\frac{AB}{2\sqrt3} = \frac{\sqrt3}{2}\) |
| বা, \(AB = \frac{2\sqrt3 \times \sqrt3}{2}\) |
| বা, \(AB = 3\) মিটার. |
2. ABC সমকোণী ত্রিভুজের ∠B সমকোণ। AB = 8√3 সেমি. এবং BC = 8সেমি. হলে, ∠ACB ও ∠BAC-এর মান হিসাব করে লিখি।
সমাধানঃ-
সমকোণী ▲ABC তে,
| \(\tan \angle ACB = \frac{AB}{BC}\) |
| বা, \(\tan \angle ACB = \frac{8\sqrt3}{8}\) |
| বা, \(\tan \angle ACB = \sqrt3\) |
| বা, \(\tan \angle ACB = \tan60^°\) |
| বা, \(\angle ACB = 60^°\) |
আবার,
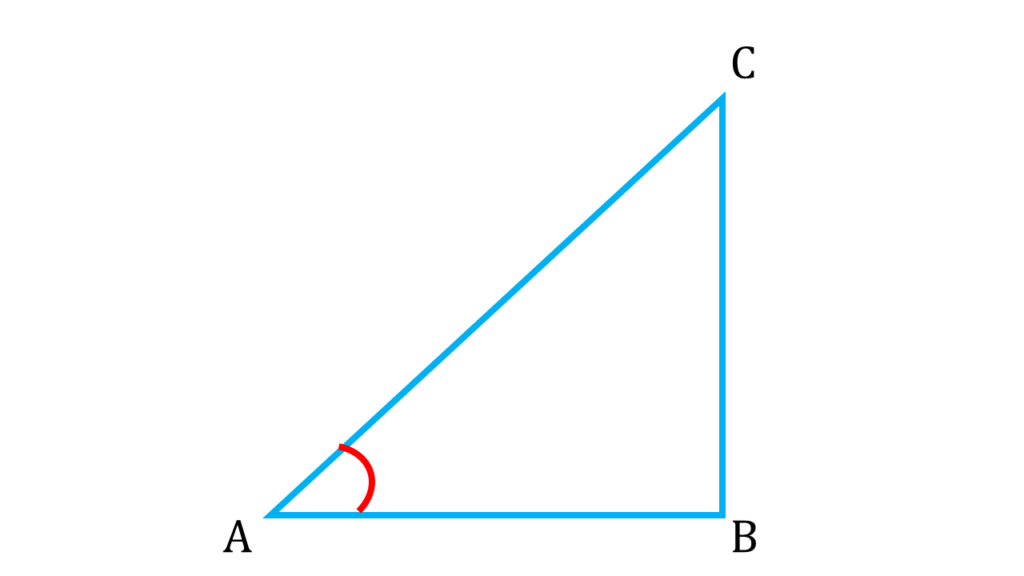
| \(\tan \angle BAC = \frac{BC}{AB}\) |
| বা, \(\tan \angle BAC = \frac{8}{8\sqrt3}\) |
| বা, \(\tan \angle BAC = \frac{1}{\sqrt3}\) |
| বা, \(\tan \angle BAC = \tan 30^°\) |
| বা, \(\angle BAC = 30^°\) |
3. ABC সমকোণী ত্রিভুজের ∠B = 90°, ∠A = 30° এবং AC = 20 সেমি.। BC এবং AB বাহুদ্বয়েরদৈর্ঘ্য হিসাব করে লিখি।
সমাধানঃ-
সমকোণী ▲ABC তে,
| \(\frac{BC}{AC} = \sin 30^°\) |
| বা, \(\frac{BC}{20} = \frac{1}{2}\) |
| বা, \(BC = \frac{20}{2}\) |
| বা, \(BC = 10\) সেমি. |
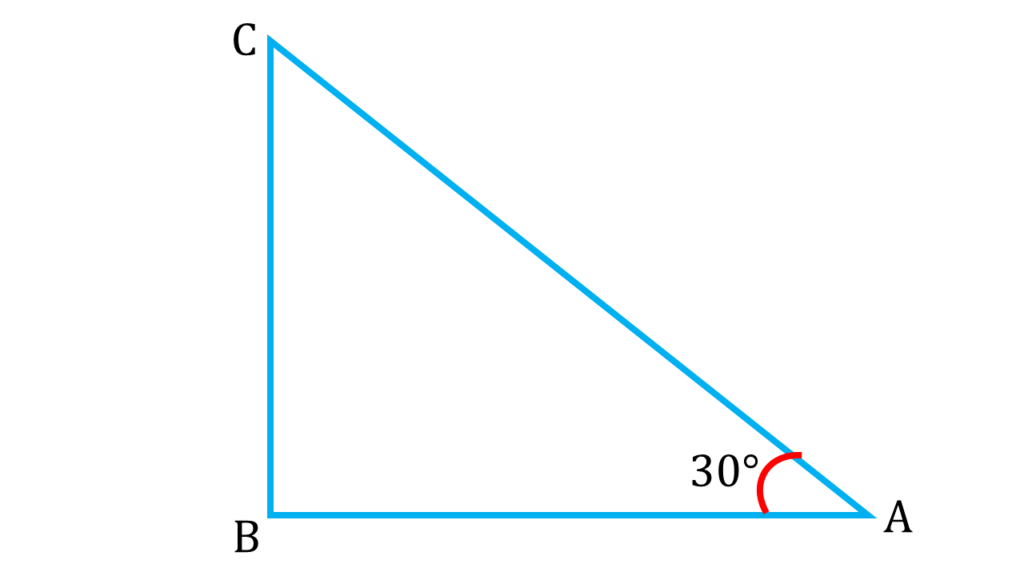
আবার,
| \(\frac{AB}{AC} = \cos 30^°\) |
| বা, \(\frac{AB}{20} = \frac{\sqrt3}{2}\) |
| বা, \(AB = \frac{20\sqrt3}{2}\) |
| বা, \(AB = 10\sqrt3\) সেমি. |
4. PQR সমকোণী ত্রিভুজের ∠Q = 90°, ∠R = 45° ; যদি PR = 3√2 মিটার হয়, তাহলে PQ ও QR বাহুদ্বয়ের দৈর্ঘ্য নির্ণয় করি।
সমাধানঃ-
যেহেতু PQR সমকোণী ত্রিভুজের ∠Q = 90°, ∠R = 45° সেহেতু ∠P = 90° – 45° = 45°
সুতরাং, PQ = QR

PQR সমকোণী ত্রিভুজে
| \(\frac{PQ}{PR} = \sin 45^°\) |
| বা, \(\frac{PQ}{3\sqrt2} = \frac{1}{\sqrt2}\) |
| বা, \(PQ = \frac{3\sqrt2}{\sqrt2}\) |
| বা, \(PQ = 3 = QR\) মিটার. |
5. মান নির্ণয় করি :
(i) sin245°- cosec260°+ sec230°
সমাধানঃ-
| sin245°- cosec260°+ sec230° |
| \(= (\frac{1}{\sqrt2})^2 – (\frac{2}{\sqrt3})^2 + (\frac{2}{\sqrt3})^2\) |
| \(= \frac{1}{2} – \frac{4}{3} + \frac{4}{3}\) |
| \(= \frac{1}{2}\) |
(ii) sec245°- cot245°- sin2 30° – sin260°
সমাধানঃ-
| sec245°- cot245°- sin2 30° – sin260° |
| \(= (\sqrt2)^2 – (1)^2 – (\frac{1}{2})^2 – (\frac{\sqrt3}{2})^2\) |
| \(= 2 – 1 – \frac{1}{4} – \frac{3}{4}\) |
| \(= 1 – \frac{1}{4} – \frac{3}{4}\) |
| \(= \frac{4 – 1 – 3}{4}\) |
| \(= \frac{0}{4}\) |
| \(= 0\) |
(iii) 3tan245° – sin260° – \(\frac{1}{3}\)cot230° – \(\frac{1}{8}\)sec245°
সমাধানঃ-
| 3tan245° – sin260° – \(\frac{1}{3}\)cot230° – \(\frac{1}{8}\)sec245° |
| \(= 3(1)^2 – (\frac{\sqrt3}{2})^2 – \frac{1}{3}(\frac{1}{\sqrt3})^2 – \frac{1}{8} (\sqrt2)^2\) |
| \(= 3 – \frac{3}{4} – \frac{1}{3} \times \frac{1}{3} – \frac{1}{8} \times 2\) |
| \(= 3 – \frac{3}{4} – \frac{1}{9} – \frac{1}{4}\) |
| \(= \frac{3 \times 36 – 3 \times 9 – 9 \times 4 – 9}{36}\) |
| \(= \frac{108 – 27 – 36 – 9}{36}\) |
| \(= \frac{36}{36}\) |
| \(= 1\) |
(iv) \(\frac{4}{3}\cot^230^° + 3\sin^260^° – 2\csc^260^° – \frac{3}{4}\tan^230^°\)
সমাধানঃ-
| \(\frac{4}{3}\cot^230^° + 3\sin^260^° – 2\csc^260^° – \frac{3}{4}\tan^230^°\) |
| \(= \frac{4}{3} \times (\sqrt3)^2 + 3 \times (\frac{\sqrt3}{2})^2 – 2 \times (\frac{2}{\sqrt3})^2 – \frac{3}{4} \times (\frac{1}{\sqrt3})^2\) |
| \(= \frac{4}{3} \times 3 + 3 \times (\frac{3}{4} – 2 \times \frac{4}{3} – \frac{3}{4} \times \frac{1}{3}\) |
| \(= 4 + \frac{9}{4} – \frac{8}{3} – \frac{1}{4}\) |
| \(= \frac{4 \times 12 + 9 \times 3 – 8 \times 4 – 3}{12}\) |
| \(= \frac{48 + 27 – 32 – 3}{12}\) |
| \(= \frac{40}{12}\) |
| \(= \frac{10}{3}\) |
| \(= 3\frac{1}{3}\) |
(v) \(\frac{\frac{1}{3}\cos30^°}{\frac{1}{2}\sin45^°} + \frac{\tan60^°}{\cos30^°}\)
সমাধানঃ-
| \(\frac{\frac{1}{3}\cos30^°}{\frac{1}{2}\sin45^°} + \frac{\tan60^°}{\cos30^°}\) |
| \(= \frac{\frac{1}{3} \times \frac{\sqrt3}{2}}{\frac{1}{2} \times \frac{1}{\sqrt2}} + \frac{\sqrt3}{\frac{\sqrt3}{2}}\) |
| \(= \frac{\sqrt6}{3} + 2\) |
| \(= \frac{\sqrt6 + 6}{3}\) |
(vi) cot230° – 2cos260° – \(\frac{3}{4}\)sec245° – 4sin230°
সমাধানঃ-
| cot230° – 2cos260° – \(\frac{3}{4}\)sec245° – 4sin230° |
| \(= (\sqrt3)^2 – 2 \times (\frac{1}{2})^2 – \frac{3}{4} \times (\sqrt2)^2 – 4 \times (\frac{1}{2})^2\) |
| \(= 3 – 2 \times \frac{1}{4} – \frac{3}{4} \times 2 – 4 \times \frac{1}{4}\) |
| \(= 3 – \frac{1}{2} – \frac{3}{2} – 1\) |
| \(= 2 – \frac{1+3}{2}\) |
| \(= 2 – \frac{4}{2}\) |
| \(= 2 – 2\) |
| \(= 0\) |
(vii) \(\sec^260^° – \cot^230° – \frac{2\tan30^° \csc60^°}{1 + \tan^230^°}\)
সমাধানঃ-
| \(\sec^260^° – \cot^230° – \frac{2\tan30^° \csc60^°}{1 + \tan^230^°}\) |
| \(= (2)^2 – (\sqrt3)^2 – \frac{2 \times \frac{1}{\sqrt3} \times \frac{2}{\sqrt3}}{1 + (\frac{1}{\sqrt3})^2}\) |
| \(= 4 – 3 – \frac{\frac{4}{3}}{1 + \frac{1}{3}}\) |
| \(= 1 – \frac{\frac{4}{3}}{\frac{3+1}{3}}\) |
| \(= 1 – \frac{\frac{4}{3}}{\frac{4}{3}}\) |
| \(= 1 – 1 = 0\) |
(viii) \( \frac{\tan60^° – \tan30^° }{1 + \tan60^° \tan30^°} + \cos60^° \cos30^° + \sin 60^°\sin 30^°\)
সমাধানঃ-
| \( \frac{\tan60^° – \tan30^° }{1 + \tan60^° \tan30^°} + \cos60^° \cos30^° + \sin 60^°\sin 30^°\) |
| \(= \frac{\sqrt3 – \frac{1}{\sqrt3}}{1 + \sqrt3 \times \frac{1}{\sqrt3}} + \frac{1}{2} \times \frac{\sqrt3}{2} + \frac{\sqrt3}{2} \times \frac{1}{2}\) |
| \(= \frac{ \frac{3 – 1}{\sqrt3}}{1 + 1} + \frac{\sqrt3}{4} + \frac{\sqrt3}{4}\) |
| \(= \frac{1}{\sqrt3} + \frac{\sqrt3}{2}\) |
| \(= \frac{2 + 3}{2\sqrt3}\) |
| \(= \frac{5}{2\sqrt3}\) |
(ix) \(\frac{1 – \sin^230^°}{1 + \sin^245^°} \times \frac{\cos^260^° + \cos^230^°}{\csc^290^° – \cot^290^°} ÷ (\sin60^°\tan30^°)\)
সমাধানঃ-
| \(\frac{1 – \sin^230^°}{1 + \sin^245^°} \times \frac{\cos^260^° + \cos^230^°}{\csc^290^° – \cot^290^°} ÷ (\sin60^°\tan30^°)\) |
| \(= \frac{1 – (\frac{1}{2})^2}{1 + (\frac{1}{\sqrt2})^2} \times \frac{(\frac{1}{2})^2 + (\frac{\sqrt3}{2})^2}{1^2 – 0} ÷ (\frac{\sqrt3}{2} \times \frac{1}{\sqrt3})\) |
| \(= \frac{1 – \frac{1}{4}}{1 + \frac{1}{2}} \times \frac{\frac{1}{4} + \frac{3}{4}}{1} ÷ \frac{1}{2}\) |
| \(= \frac{\frac{3}{4}}{\frac{3}{2}} \times 1 \times 2\) |
| \(= 1\) |
6. দেখাই যে,
(i) \(\sin^245^° + \cos^245^° = 1\)
সমাধানঃ-
| \(\sin^245^° + \cos^245^°\) |
| \(= (\frac{1}{\sqrt2})^2 + (\frac{1}{\sqrt2})^2\) |
| \(= \frac{1}{2} + \frac{1}{2}\) |
| \(= 1 \) |
(ii) \(\cos60^° = \cos^230^° – \sin^230^°\)
সমাধানঃ-
| \(\cos^230^° – \sin^230^°\) |
| \(= (\frac{\sqrt3}{2})^2 – (\frac{1}{2})^2\) |
| \(= \frac{3}{4} – \frac{1}{4}\) |
| \(= \frac{3-1}{4}\) |
| \(= \frac{2}{4}\) |
| \(= \frac{1}{2}\) |
| \(= \cos60^°\) |
(iii) \(\frac{2\tan30^°}{1 – \tan^230^°} = \sqrt{3}\)
সমাধানঃ-
| \(\frac{2\tan30^°}{1 – \tan^230^°} \) |
| \(= \frac{2 \times \frac{1}{\sqrt3}}{1 – (\frac{1}{\sqrt3})^2} \) |
| \(= \frac{2 \times \frac{1}{\sqrt3}}{1 – \frac{1}{3}} \) |
| \(= \frac{2 \times \frac{1}{\sqrt3}}{ \frac{3-1}{3}} \) |
| \(= \frac{\frac{2}{\sqrt3}}{ \frac{2}{3}} \) |
| \(= \frac{3}{\sqrt3}\) |
| \(= \sqrt3\) |
(iv) \(\sqrt{\frac{1 + \cos30^°}{1 – \cos30^°}} = \sec60^° + \tan60^°\)
সমাধানঃ-
| L.H.S |
|---|
| \(\sqrt{\frac{1 + \cos30^°}{1 – \cos30^°}}\) |
| \(= \sqrt{\frac{1 + \frac{\sqrt3}{2}}{1 – \frac{\sqrt3}{2}}}\) |
| \(= \sqrt{\frac{\frac{2+\sqrt3}{2}}{\frac{2 – \sqrt3}{2}}}\) |
| \(= \sqrt{\frac{2 + \sqrt3}{2 – \sqrt3}}\) |
| \(= \sqrt{\frac{(2 + \sqrt3)(2 + \sqrt3)}{2^2 – (\sqrt3)^2}}\) |
| \(= \sqrt{\frac{(2 + \sqrt3)^2}{4 – 3}}\) |
| \(= 2 + \sqrt3\) |
| R.H.S |
|---|
| \(\sec60^° + \tan60^°\) |
| \(=2 + \sqrt3\) |
সুতরাং,
\(\sqrt{\frac{1 + \cos30^°}{1 – \cos30^°}} = \sec60^° + \tan60^°\)
(v) \(\frac{2\tan^230^°}{1 – \tan^230^°} + \sec^245^° – \cot^245^° = \sec60^°\)
সমাধানঃ-
| \(\frac{2\tan^230^°}{1 – \tan^230^°} + \sec^245^° – \cot^245^°\) |
| \(= \frac{2 \times (\frac{1}{\sqrt3})^2}{1 – (\frac{1}{\sqrt3})^2} + (\sqrt2)^2 – 1^2\) |
| \(= \frac{\frac{2}{3}}{1 – \frac{1}{3}} + 2 – 1\) |
| \(= \frac{\frac{2}{3}}{\frac{3-1}{3}} + 2 – 1\) |
| \(= \frac{\frac{2}{3}}{\frac{2}{3}} + 1\) |
| \(= 1 + 1\) |
| \(= 2 = \sec60^°\) |
(vi) \(\tan^2\frac{π}{4} \sin\frac{π}{3} \tan\frac{π}{6} \tan^2\frac{π}{3} = 1\frac{1}{2}\)
সমাধানঃ-
| \(\tan^2\frac{π}{4} \sin\frac{π}{3} \tan\frac{π}{6} \tan^2\frac{π}{3}\) |
| \(= \tan^245^° \sin60^° \tan30^° \tan^260^°\) |
| \(= 1^2 \times \frac{\sqrt3}{2} \times \frac{1}{\sqrt3} \times (\sqrt3)^2\) |
| \(= \frac{1}{2} \times 3\) |
| \(= \frac{3}{2}\) |
| \(= 1\frac{1}{2}\) |
(vii) \(\sin\frac{π}{3} \tan\frac{π}{6} + \sin\frac{π}{2} \cos\frac{π}{3} = 2\sin^2\frac{π}{4}\)
সমাধানঃ-
| \(\sin\frac{π}{3} \tan\frac{π}{6} + \sin\frac{π}{2} \cos\frac{π}{3}\) |
| \(= \sin60^° \tan30^° + \sin90^° \cos60^°\) |
| \(= \frac{\sqrt3}{2} \times \frac{1}{\sqrt3} + 1 \times \frac{1}{2}\) |
| \(= \frac{1}{2} + \frac{1}{2}\) |
| \(= 2 \times \frac{1}{2}\) |
| \(= 2 \times (\frac{1}{\sqrt2})^2\) |
| \(= 2\sin^245^°\) |
| \(= 2\sin^2\frac{π}{4}\) |
7.
(i) \(x\sin45^° \cos45^° \tan60^° = \tan^245^° – \cos60^°\) হলে, \(x\) এর মান নির্ণয় করি।
সমাধানঃ-
| \(x\sin45^° \cos45^° \tan60^° = \tan^245^° – \cos60^°\) |
| বা, \(x \times \frac{1}{\sqrt2} \times \frac{1}{\sqrt2} \times \sqrt3 = 1^2 – \frac{1}{2}\) |
| বা, \(x \times \frac{\sqrt3}{2} = \frac{1}{2}\) |
| বা, \(x = \frac{2}{\sqrt3 \times 2}\) |
| বা, \(x = \frac{1}{\sqrt3}\) |
(ii) \(x\sin60^° \cos^2 30^° = \frac{\tan^245^° \sec60^°}{\csc60^°}\) হলে, \(x\) এর মান নির্ণয় করি।
সমাধানঃ-
| \(x\sin60^° \cos^2 30^° = \frac{\tan^245^° \sec60^°}{\csc60^°}\) |
| বা, \(x \times \frac{\sqrt3}{2} \times (\frac{\sqrt3}{2})^2 = \frac{1^2 \times 2}{\frac{2}{\sqrt3}}\) |
| বা, \(x \times \frac{3\sqrt3}{8} = \sqrt3\) |
| বা, \(x = \frac{8}{3}\) |
| বা, \(x = 2\frac{2}{3}\) |
(iii) \(x^2 = \sin^230^° + 4\cot^245^° – \sec^260^°\) হলে, \(x\) এর মান নির্ণয় করি।
সমাধানঃ-
| \(x^2 = \sin^230^° + 4\cot^245^° – \sec^260^°\) |
| বা, \(x^2 = (\frac{1}{2})^2 + 4\times 1^2 – 2^2\) |
| বা, \(x^2 = \frac{1}{4} + 4 – 4\) |
| বা, \(x = \pm \frac{1}{2}\) |
8. \(x\tan30^° + y\cot60^° = 0\) এবং \(2x – y\tan45^° = 1\) হলে, \(x\) ও \(y\) -এর মান হিসাব করে লিখি।
সমাধানঃ-
| \(x\tan30^° + y\cot60^° = 0\) |
| বা, \(x \times \frac{1}{\sqrt3} + y \times \frac{1}{\sqrt3} = 0\) |
| বা, \(x + y = 0\) |
| বা, \(y = – x\) ——–(i) |
| \(2x – y\tan45^° = 1\) |
| বা, \(2x – y = 1\) |
| বা, \(2x – (-x) = 1\) [(i) নং থেকে y এর মান বসিয়ে পাই] |
| বা, \(2x + x = 1\) |
| বা, \(3x = 1\) |
| বা, \(x = \frac{1}{3}\) |
(i) নং সমীকরণে \(x\) এর মান বসিয়ে পাই,
| \(y = – \frac{1}{3}\) |
9. যদি \(A = B = 45^°\) হয়, তবে যাচাই করি যে,
(i) \(\sin (A+B) = \sin A \cos B + \cos A \sin B\)
সমাধানঃ-
| \(\sin A \cos B + \cos A \sin B\) |
| \(= \sin 45^° \cos 45^° + \cos 45^° \sin 45^°\)\) |
| \(= \frac{1}{\sqrt2} \times \frac{1}{\sqrt2} + \frac{1}{\sqrt2} \times \frac{1}{\sqrt2}\) |
| \(= \frac{1}{2} + \frac{1}{2}\) |
| \(= 1\) |
| \(= \sin 90^°\) |
| \(= \sin(45^° + 45^°)\) |
| \(= \sin (A + B)\) |
(ii) \(\cos (A+B) = \cos A \cos B – \sin A \sin B\)
সমাধানঃ-
| \(\cos A \cos B – \sin A \sin B\) |
| \(= \cos 45^° \cos 45^° – \sin 45^° \sin 45^°\)\) |
| \(= \frac{1}{\sqrt2} \times \frac{1}{\sqrt2} – \frac{1}{\sqrt2} \times \frac{1}{\sqrt2}\) |
| \(= \frac{1}{2} – \frac{1}{2}\) |
| \(= 0\) |
| \(= \cos 90^°\) |
| \(= \cos(45^° + 45^°)\) |
| \(= \cos (A + B)\) |
10.
(i) ABC সমবাহু ত্রিভুজের BD একটি মধ্যমা। প্রমাণ করি যে, \(\tan \angle ABD = \cot \angle BAD\)
সমাধানঃ-
ABC সমবাহু ত্রিভুজের BD একটি মধ্যমা।
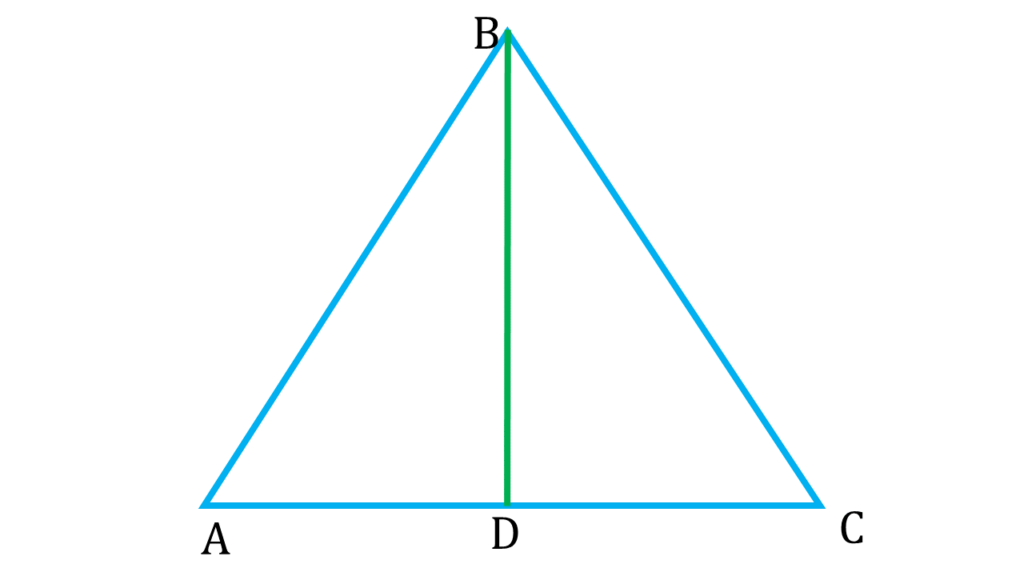
অতএব, BD⊥AC
আবার,
▲ABD সমকোণী ত্রিভুজ এবং ∠BAD = 60° [∵ ABC সমবাহু ত্রিভুজ]
অতএব, ∠ABD = 30°
এখন, \(\tan \angle ABD = \tan30^° = \frac{1}{\sqrt3}\)
এবং
\(\cot \angle BAD = \cot60^° = \frac{1}{\sqrt3}\)
সুতরাং,
\(\tan \angle ABD = \cot \angle BAD\)
(ii) ABC সমদ্বিবাহু ত্রিভুজের AB = AC এবং \(\angle BAC = 90^°\) ; \(\angle BAC\)-এর সমদ্বিখন্ডক BC বাহুকে D বিন্দুতে ছেদ করে।
প্রমাণ করি যে,
\(\frac{\sec\angle ACD}{\sin\angle CAD} = \csc^2 \angle CAD\)
সমাধানঃ-
ABC সমদ্বিবাহু ত্রিভুজের AB = AC এবং \(\angle BAC = 90^°\) ; \(\angle BAC\)-এর সমদ্বিখন্ডক BC বাহুকে D বিন্দুতে ছেদ করে।
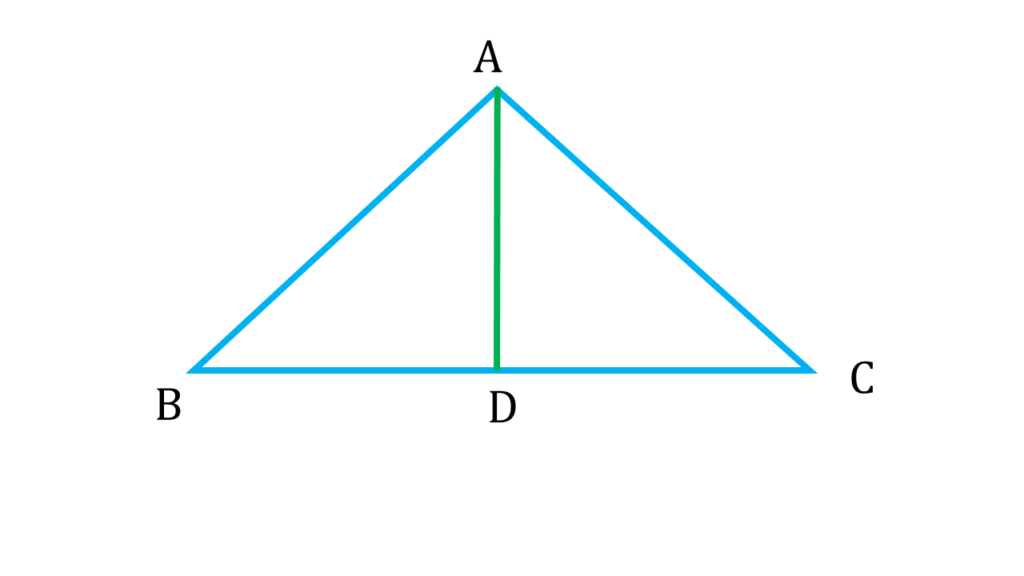
অতএব, \(\angle ABC = \angle ACB = 45^°\)
সুতরাং, \(\angle CAD = 45^°\)
| L.H.S |
|---|
| \(\frac{\sec\angle ACD}{\sin\angle CAD}\) |
| \(= \frac{\sec45^°}{\sin45^°}\) |
| \(= \frac{\sqrt2}{\frac{1}{\sqrt2}}\) |
| \(= 2\) |
| R.H.S |
|---|
| \(\csc^2 \angle CAD\) |
| \(= \csc^245^°\) |
| \(= (\sqrt2)^2\) |
| \(= 2\) |
সুতরাং,
\(\frac{\sec\angle ACD}{\sin\angle CAD} = \csc^2 \angle CAD\)
11. \(\theta (0^° \leq \theta \leq 90^°)\) – এর কোন মান / মানগুলির জন্য \(2\cos^2 \theta – 3\cos \theta + 1 = 0\) সত্য হবে নির্ণয় করি।
সমাধানঃ-
| \(2\cos^2 \theta – 3\cos \theta + 1 = 0\) |
| বা, \(2\cos^2 \theta – \cos \theta – 2\cos \theta + 1 = 0\) |
| বা, \(\cos \theta(2\cos \theta – 1) – (2\cos \theta – 1) = 0\) |
| বা, \((2\cos \theta – 1)(\cos \theta – 1) = 0\) |
অতএব,
\(2\cos \theta – 1 = 0\)
বা, \(\cos \theta = \frac{1}{2}\)
বা, \(\cos \theta = \cos60^°\)
বা, \(\theta = 60^°\)
এবং,
\(\cos \theta – 1 = 0\)
বা, \(\cos \theta = 1\)
বা, \(\cos \theta = \cos0^°\)
বা, \(\theta = 0^°\)
Class 10 WBBSE এর বাকি অধ্যায়ের সমাধান-
| অধ্যায় | সমাধান |
| 1. একচলবিশিষ্ট দ্বিঘাত সমীকরণ (Quadratic Equations with one variable) | কষে দেখি 1.1 |
| কষে দেখি 1.2 | |
| কষে দেখি 1.3 | |
| কষে দেখি 1.4 | |
| কষে দেখি 1.5 | |
| 2. সরল সুদকষা (Simple Interest) | কষে দেখি 2 |
| 3. বৃত্ত সম্পর্কিত উপপাদ্য (Theorems related to circle) | কষে দেখি 3.1 |
| কষে দেখি 3.2 | |
| 4. আয়তঘন (Rectangular Parallelopiped or Cuboid) | কষে দেখি 4 |
| 5. অনুপাত ও সমানুপাত ( Ratio and Proportion) | কষে দেখি 5.1 |
| কষে দেখি 5.2 | |
| কষে দেখি 5.3 | |
| 6. চক্রবৃদ্ধি সুদ ও সমহার বৃদ্ধি বা হ্রাস (Compound Interest and Uniform Rate of Increase or Decrease) | কষে দেখি 6.1 |
| কষে দেখি 6.2 | |
| 7. বৃত্তস্থ কোণ সম্পর্কিত উপপাদ্য (Theorems related to Angles in a Circle) | কষে দেখি 7.1 |
| কষে দেখি 7.2 | |
| কষে দেখি 7.3 | |
| 8. লম্ব বৃত্তাকার চোঙ (Right Circular Cylinder) | কষে দেখি 8 |
| 9. দ্বিঘাত করণী (Quadratic Surd). | কষে দেখি 9.1 |
| কষে দেখি 9.2 | |
| কষে দেখি 9.3 | |
| 10. বৃত্তস্থ চতুর্ভুজ সংক্রান্ত উপপাদ্য (Theorems related to Cyclic Quadrilateral) | কষে দেখি 10 |
| 11. সম্পাদ্য : ত্রিভুজের পরিবৃত্ত ও অন্তবৃত্ত অঙ্কন | কষে দেখি 11 |
| 12. গোলক (Sphere) | কষে দেখি 12 |
| 13. ভেদ (Variation) | কষে দেখি 13 |
| 14. অংশীদারি কারবার (Partnership Business) | কষে দেখি 14 |
| 15. বৃত্তের স্পর্শক সংক্রান্ত উপপাদ্য (Theorems related to Tangent to a Circle) | কষে দেখি 15.1 |
| কষে দেখি 15.2 | |
| 16. লম্ব বৃত্তাকার শঙ্কু (Right Circular Cone) | কষে দেখি 16 |
| 17. সম্পাদ্য : বৃত্তের স্পর্শক অঙ্কন (Construction: Construction of Tangent to a circle) | কষে দেখি 17 |
| 18. সদৃশতা (Similarity) | কষে দেখি 18.1 |
| কষে দেখি 18.2 | |
| কষে দেখি 18.3 | |
| কষে দেখি 18.4 | |
| 19. বিভিন্ন ঘনবস্তু সংক্রান্ত বাস্তব সমস্যা (Real life Problems related to different Solid Objects) | কষে দেখি 19 |
| 20. ত্রিকোণমিতি : কোণ পরিমাপের ধারণা (Trigonometry: Concept of Measurment of Angle) | কষে দেখি 20 |
| 21. সম্পাদ্য : মধ্যসমানুপাতী নির্ণয় (Construction: Determination of Mean Proportional) | কষে দেখি 21 |
| 22. পিথাগোরাসের উপপাদ্য (Pythagoras Theorem) | কষে দেখি 22 |
| 23. ত্রিকোণমিতিক অনুপাত এবং ত্রিকোণমিতিক অভেদাবলি (Trigonometric Ratios and Trigonometric Identities) | কষে দেখি 23.1 |
| কষে দেখি 23.2 | |
| কষে দেখি 23.3 | |
| 24. পূরক কোণের ত্রিকোণমিতিক অনুপাত (Trigonometric Ratios of Complementrary angle) | কষে দেখি 24 |
| 25. ত্রিকোণমিতিক অনুপাতের প্রয়োগ : উচ্চতা ও দূরত্ব (Application of Trigonometric Ratios : Heights & Distances) | কষে দেখি 25 |
| 26. রাশিবিজ্ঞান : গড়, মধ্যমা, ওজাইভ, সংখ্যাগুরুমান (Statistics: Mean, Median, Ogive, Mode) | কষে দেখি 26.1 |
| কষে দেখি 26.2 | |
| কষে দেখি 26.3 | |
| কষে দেখি 26.4 |
| Request For Share |
|---|
| সমাধান গুলি ভালো লাগলে অবশ্যই বন্দুদের সাথে share করবে। নিজে শেখো ও অপরকে শিখতে সাহায্য করো। Let’s Study Together………….  |
এই কষে দেখি 23.2 Class 10|Koshe Dekhi 23.2 Class 10 এর সমাধান গুলি ভালো লাগলে অবশ্যই বন্ধুদের সাথে share করবে এবং wbstudyhub.in এই ওয়েবসাইট কে বুকমার্ক করে রাখবে যাতে যে কোনো অধ্যায়ের অংক আটকালে তোমরা তা এখানে এসে দেখে নিতে পারবে।

এখানে তোমরা তোমাদের দশম শ্রেণীতে| Class 10 এ কি কি পড়ানো হয়, মানে তোমাদের দশম শ্রেণীতে| Class 10 সিলেবাসে কি আছে তা জানার জন্যে তোমরা তোমাদের শ্রেণীর সিলেবাস এখানে দেখে নিতে পারবে ।

